Complex Circuits
Key points
- Complex circuits have connections in both series and parallel.
- Solve for current and voltage by combine resistors and finding their equivalent resistance.
Complex Circuits combine their components in a more complicated arrangement:
- Connections are be in both series in parallel.
- They can be part of multi-loop circuits.
- Often, you will have to solve for current or voltage.
- Solve for these by finding the overall equivalent resistance, using the equations for equivalent resistance in both series and parallel.
- In the example to the right, the 3 and 5 ohm resistors are combined in series, and then the resultant resistor is combined with the 24 ohm resistor in parallel.
- Solve for these by finding the overall equivalent resistance, using the equations for equivalent resistance in both series and parallel.
- Simplifying these circuits is the best way to solve for them
- This requires lots of practice

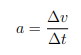
The Kinematic Equations are the same – but used for each component (vertical/horizontal)
- If velocity is not changing, then there is no acceleration
- Acceleration is a vector quantity
Suppose we choose a time interval from t = 0 to some arbitrary time t later.
Let the velocity at time t = 0 (initial velocity) be u and the velocity at time t be v (final velocity). Then we get the following expression for acceleration:
Not in Formula Booklet but important
Formula Booklet
- Rewriting the expression above leaves us with the formula booklet expression.
- The final velocity is equal to initial velocity plus the acceleration the object experienced during a given time interval
Subscribe to the Inertia Newsletter
IB News, Covid-19 Updates, Deadlines, Tips and Tricks, and Hundreds of Free Resources are Awaiting You!
Features
- Study Notes
- Thousands of IB Questions
- Detailed Answers
- Ask-A-Question System

